COLOURS:

HARMONIC FUNCTION 2

Learning outcomes: To begin understanding key changes

Q: How do we change keys?

There is no end to answering this question but metaharmony provides a road into the infinite

Ok,

so we left off from the last page understanding that all purples and greens are dominant and subdominant to the key of C

and we had this chart:

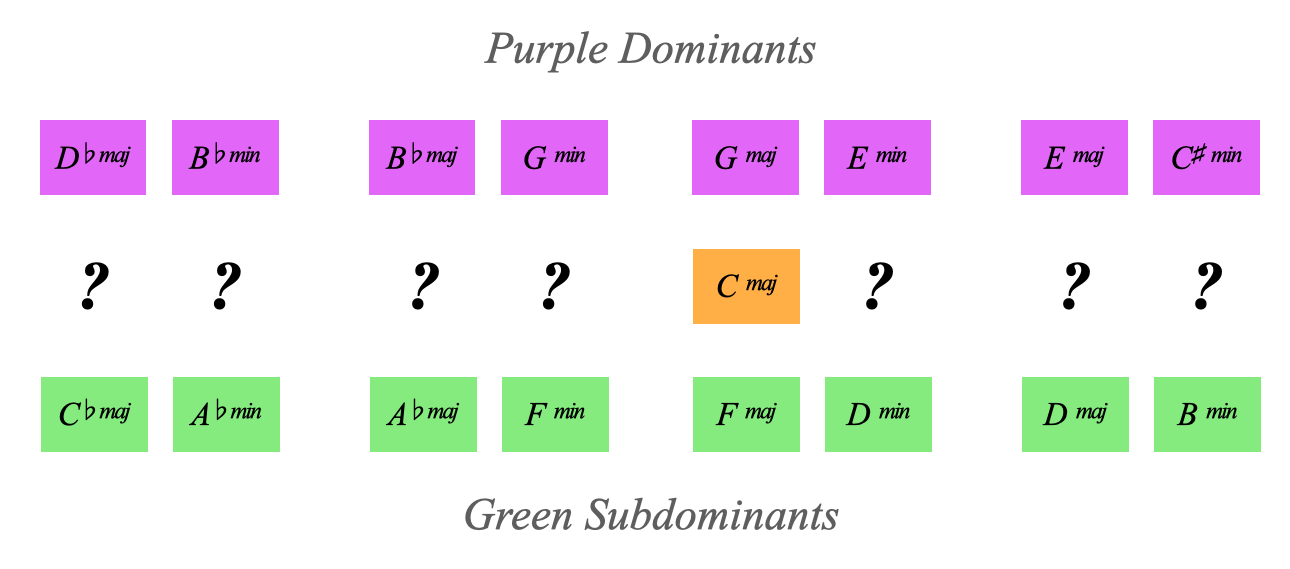
But where are all the other orange major and minor chords?

And what do they do?

They're doing two things:

1. They're providing key changes (our focus today)

2. They're providing secondary dominants (which we'll cover in one of the advanced colour pages)

If chords of the same colour have the same function,

and all purples are dominant and all greens are subdominant,

Then it follows that all oranges are tonics

But before we experience this,

We need to get more familiar with this hypercube layout:

This is a flattened out hypercube which we will understand in the geometry section of the website

But we dont need to understand the hypercube to grasp and use this chart

We can see

All the major and minor chords layed out in their colour families

But they're also organised into 4 blocks

Each block represents a key

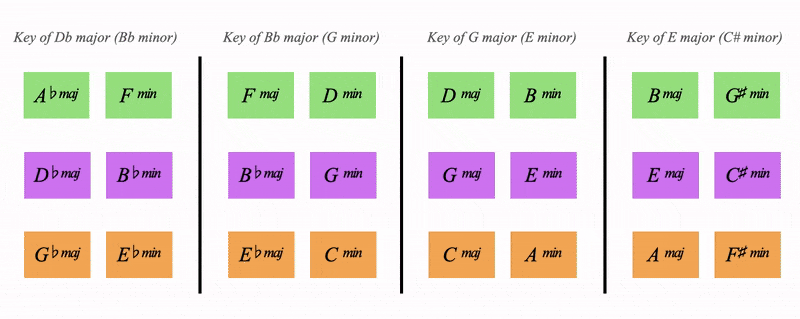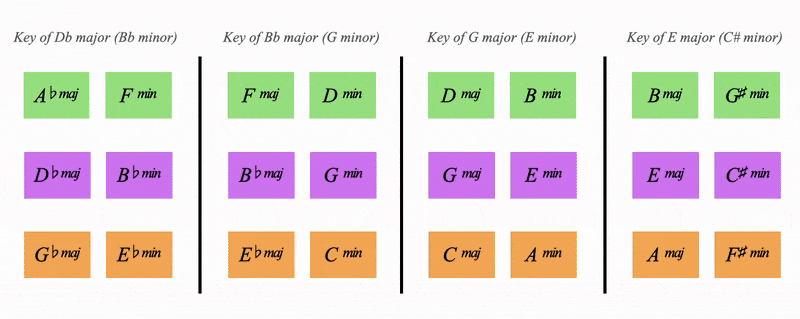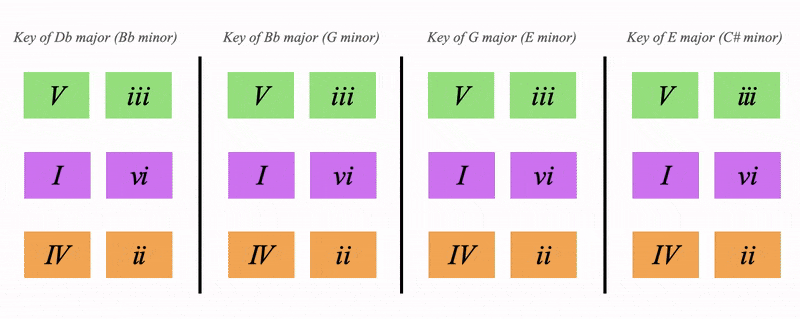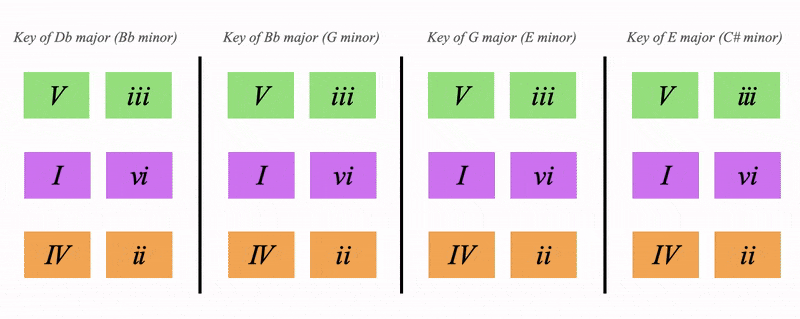
This block here has the 6 major and minor chords of C major in it, this block is the key of C major:

(2nd from the right in the chart above)

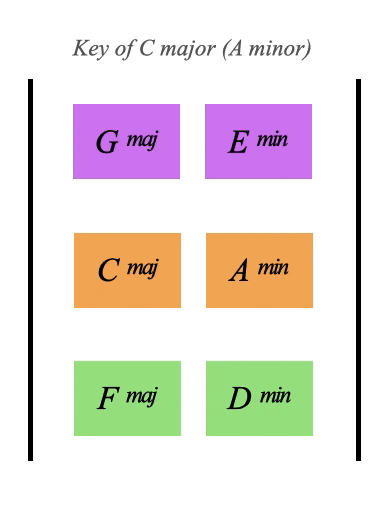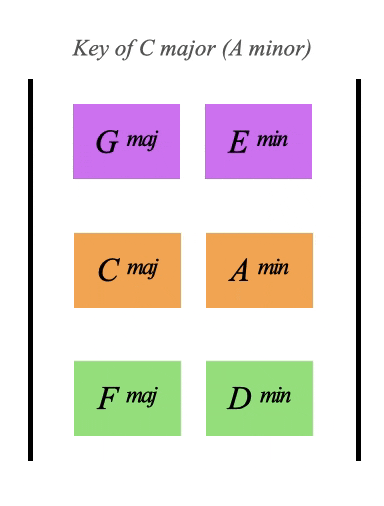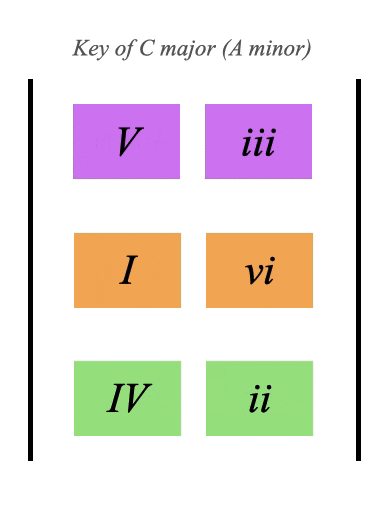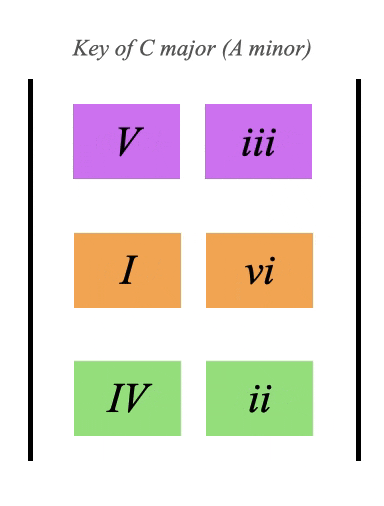
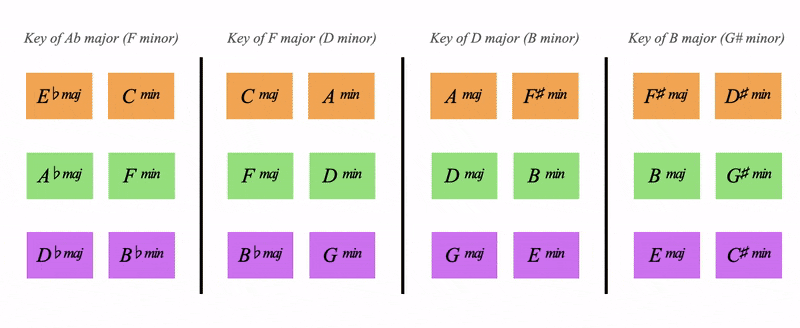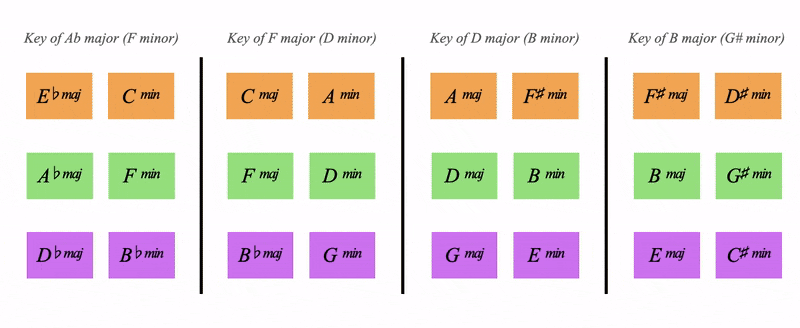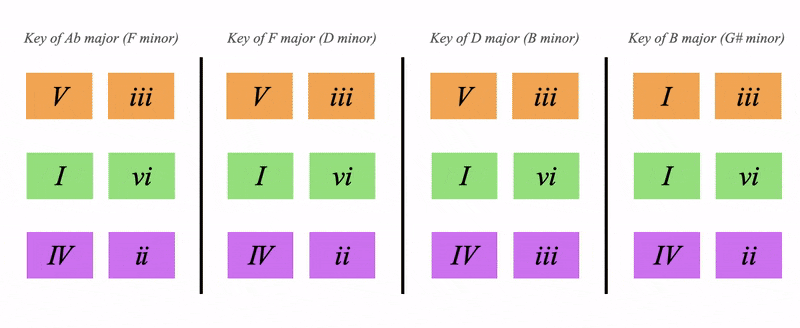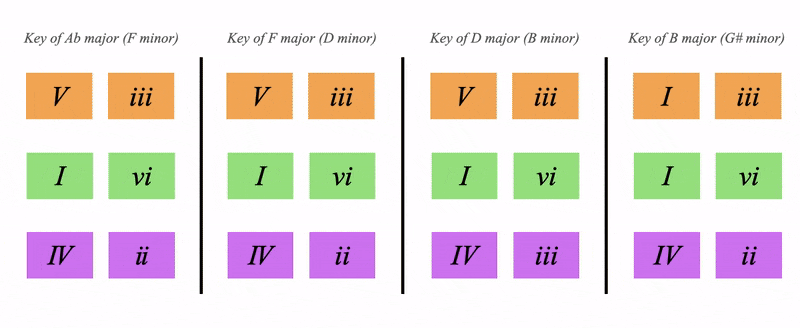
Looking back to the full chart we can see 4 keys for each of the orange chords

We have the key of C, Eb, Gb and A

Notice how the layout of the chords is the same, the V is always above the I for example

Essentially we have:

4 keys related by minor 3rds

These 4 keys share a deep relationship, they share their harmony

Ok, how?

Firstly they share all of their dominants

Remember from the last page how C major has the regular dominant (G), the tritone sub (Db), the backdoor dominant (Bb) and the dominant of minor (E)

Notice below how all 4 orange keys share these dominants

Focus on Gmaj

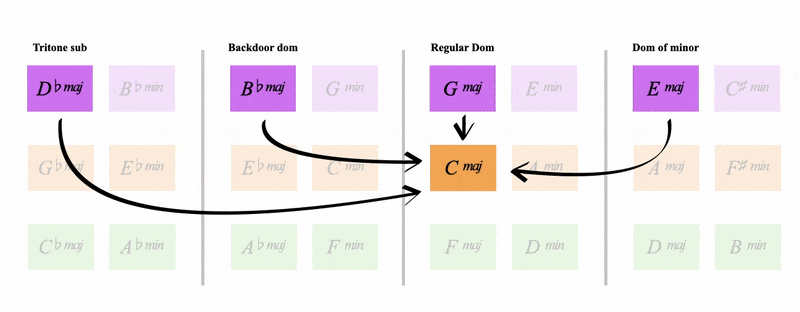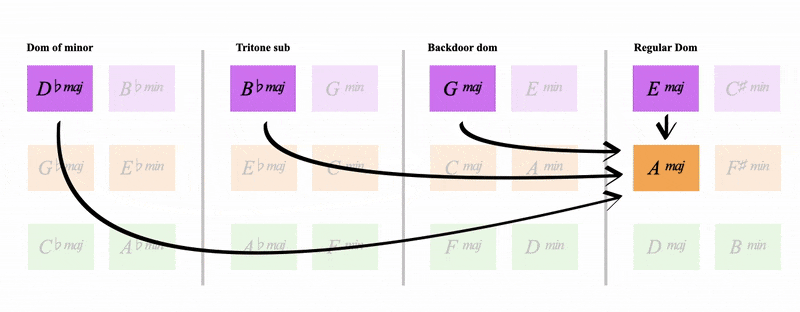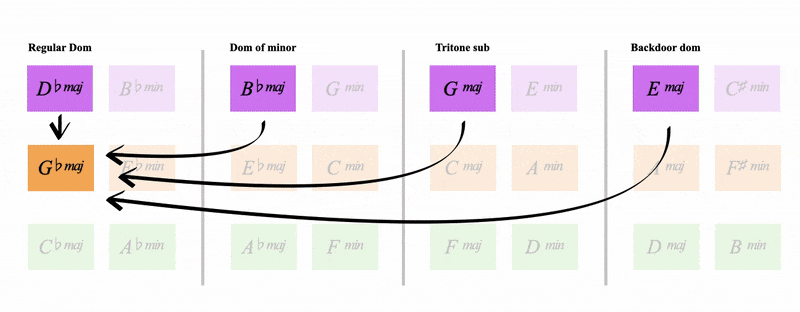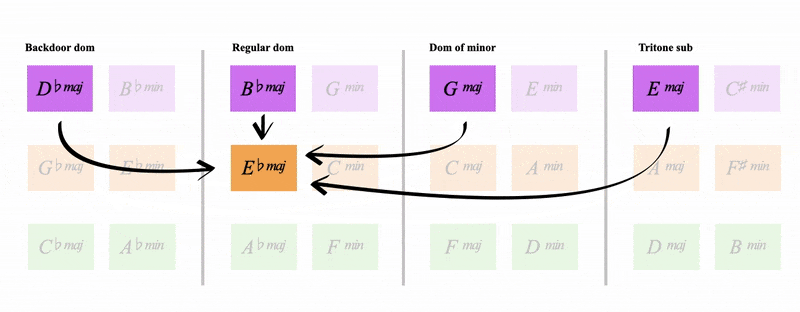
See how it's:

- The regular dominant of C major

- The backdoor dominant of A major

- The tritone sub of Gb major

- The dominant of the minor of Eb major

This harmonic sharing is also applied to the subdominants

This results in an interconnected network of orange keys

A landscape of keys that share their harmony through colour

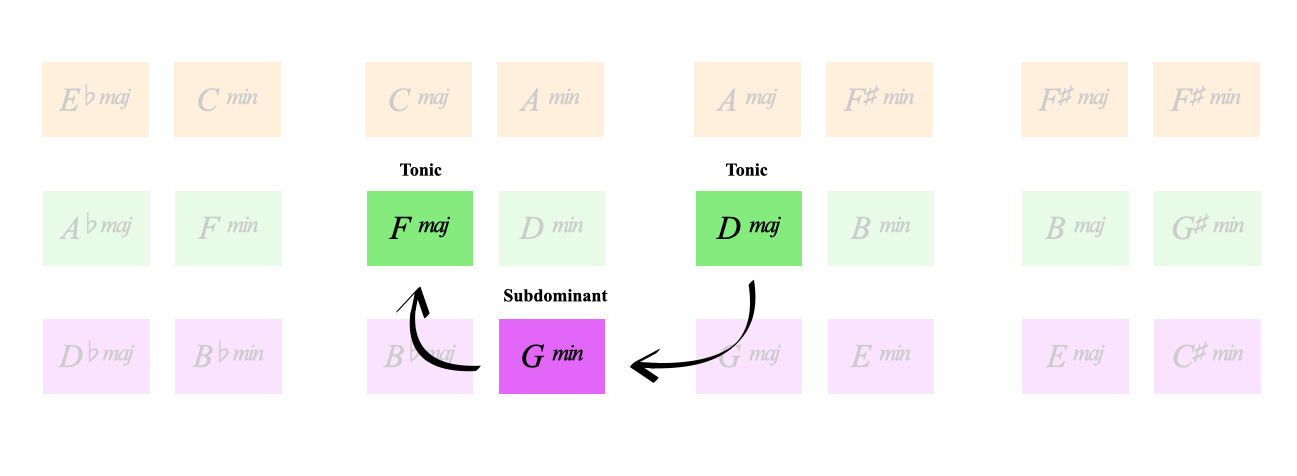
For example, F minor is the minor iv of C, but also the regular ii of Eb

We can hear the first chord as tonic, then Fm9 is the minor iv

which we resolve as ii into Eb major, we've changed key in the hypercube

How would this sound into the other orange keys?

Check out the Cook Book page on this:

So,

in the orange hypercube:

- All purples are dominant

- All greens are subdominant

- All oranges are tonics (key changes)

But what if I want to play a song thats in a green key?

What if we're playing a song in the key of Ab?

We need a green hypercube for all the green keys:

And a purple hypercube for all the purple keys:

The 3 hypercubes cover all 12 keys

and we'll dive into this more in the geometry section of the website

but this means we can do all the beautful key weaving we did in the orange hypercube

but also for green keys and purple keys

Above is the progression we did in C major (previous audio clip) but in the green hypercube starting in the key of D

Now we can play in any key by using the 3 hypercubes

"Which of the 12 keys are we in?

We're either in the orange, green or purple hypercube"

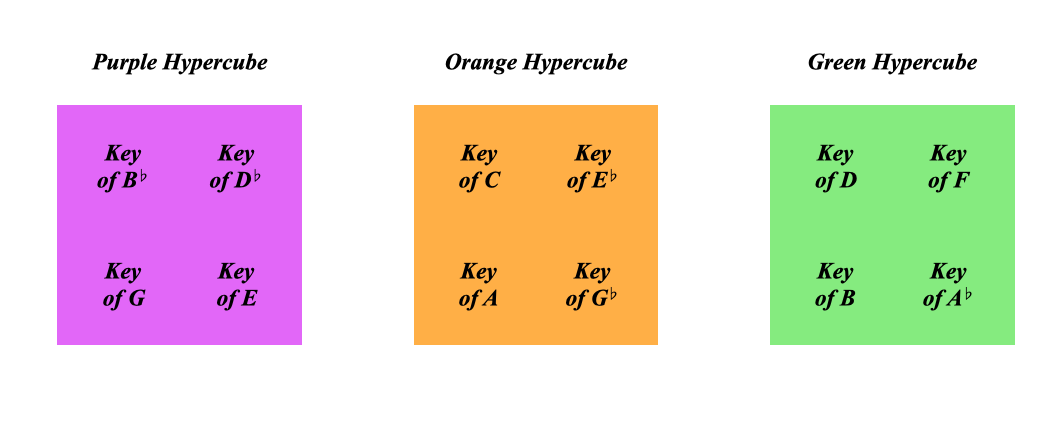
Think of it like 3 containers, each holding 4 keys

We can change between keys of the same colour by staying in one hypercube (as above)

"Let's change from the key of E to the key of Bb

We'll be moving in the purple hypercube"

There's endless ways to modulate through a hypercube (Cook Book)

But one question remains:

How do we modulate from one hypercube to another?

What if we want to go from a green key into a purple key?

We'll cover this in an advanced colour page and also in the geometry pages

and of course in the cook book

But for now we've covered the essentials of the colour system:

- Orange keys Share their dominants and subdominants

- Green keys Share their dominants and subdominants

- Purple keys Share their dominants and subdominants

Ok,

We've understood harmonic function on the colour wheel

What about chord extensions and melodic movement?

We're ready for complementary colours